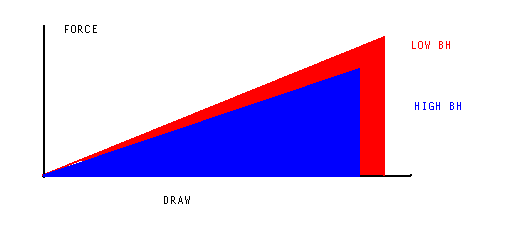
The increase in stored energy (hence higher arrow speed) for the lower bracing
height in the above example comes partly from the difference in slope of
the force draw curve and partly from the difference in draw lengths. It is a well known effect that if you increase the bracing height of a bow the arrow speed out of the bow decreases and the arrow acts 'weaker'. Decreasing the bracing height has the reverse effect. Lowering the bracing height is sometimes used therefore to get more arrow speed. Increasing the bracing height is sometimes used to 'weaken' the arrow to get over arrow clearance problems. Playing about with bracing height as part of a bow tuning process is sometimes used as it varies the effective arrow dynamic spine.
The following describes how these two effects occur. The description is based on what happens if you increase the bracing height. For a decrease in bracing height just read the following backwards. 8-)
The speed at which the arrow leaves the bow depends on how much energy is stored in the bow when you draw it and what fraction of this energy goes into the arrow's kinetic energy. Increasing the bracing height affects how much energy is stored in the bow via two mechanisms, the slope of the draw force curve and the length the arrow is drawn against the string.
As described in the section on draw force the draw force curve is determined by the spring force the limb exerts as it is bent and the string geometry at the limb and the arrow nock. As the bow is drawn the spring force exerted by the limb decreases. If you increase the the bracing height the limb starts with more bend in it and so throughout the whole of the draw the limb spring force is lower having the effect of decreasing the slope of the draw force curve. Conversely if you increase the bracing height the angles the string makes with the limb and the arrow nock are changed through the draw to act to increase the slope of the draw force curve. These two effects therefore to a large extent cancel each other out. The resulting slope of the draw force curve may be steeper, less steep or much the same depending on the limb spring properties and the length of the bow. The shorter the bow and/or the stiffer the limbs the more likely it is that the slope of the draw force curve will increase. For a 68"/70" typical modern recurve the odds are that the slope of the draw force curve will remain much the same or decrease slightly. You can easily check the draw force variation at two different brace heights with a bow scale to see what is happening with a specific bow.
If the bracing height is increased by say 1" then when the bow is drawn the arrow is drawn 1" less against the string and so there will be less energy stored in the bow.(The loss of 1" of draw over the draw force curve you had with the original bracing height).
The following diagram illustrates the overall effect. The energy stored in the bow is represented by the area under the draw force curve.
The increase in stored energy (hence higher arrow speed) for the lower bracing
height in the above example comes partly from the difference in slope of
the force draw curve and partly from the difference in draw lengths.
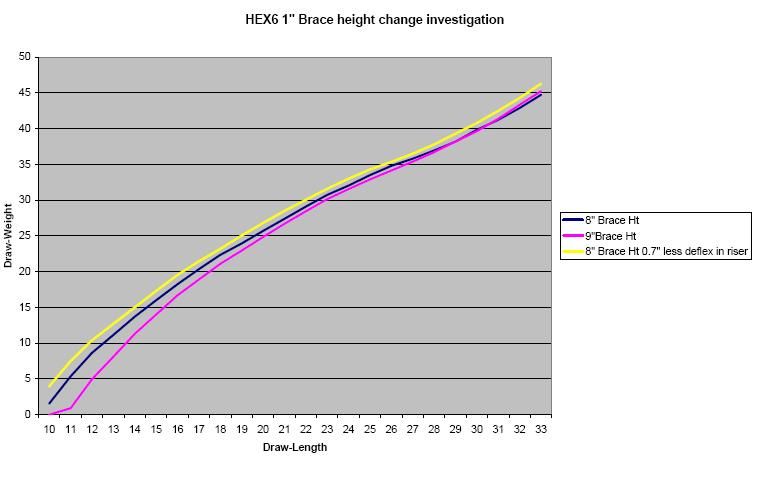Here (from Border) is a practical example of how changing the brace height affects the draw force curve and hence the stored energy. Also included
(the yellow curve) is an example of how the changing the brace height via modifying the riser (deflex) shape can be used to increase energy storage and
hence increase arrow speed. The limitation on this approach is that bow torsional stability is reduced and the limbs are more likely to stack.
Increasing the bracing height has two effects on the amount the arrow initially bends (its dynamic spine) when it is released. Firstly, as illustrated in the above diagram, it will in general lower the weight on the fingers at full draw. This will have the effect of reducing the amount the arrow bends (stiffer arrow). The second effect is that increasing the bracing height reduces the length of shaft over which this bending occurs and increasing the string force angle to the shaft which has the effect of increasing the amount the arrow bends (weaker arrow). In general the latter effect predominates and the overall effect is to 'weaken' the arrow.
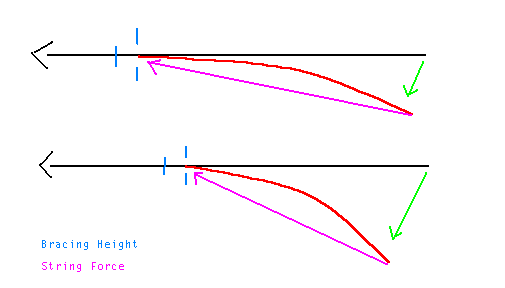How the arrow bends on release is described in the section on Archers Paradox.
The section of arrow shaft over which the 'nock end' bending occurs is from
the nock end to a point a bit short of where the bracing height is located.
( a 'bit short' as the arrow is moving forward as it bends). If you assume
that the amount of 'spring energy' that builds in the shaft until the pile
speed catches up with the nock speed is much the same for a small change
in bracing height then although the length of bent shaft is a bit shorter,
and hence stiffer, the angle between the string force and the shaft is bigger
and overall the shaft bends more with the higher bracing height. Another
way to put this is to say that as the bracing height increases a lower fraction
of the string force goes into compressing the arrow shaft and a higher fraction
into bending the arrow shaft.
The actual position of the bracing height will affect what effect the string has on the arrow at the point where the string separates from the nock. During the power stroke the bow string is accelerating, pushing the arrow. At some point, determined by the bracing height the string will start to decelerate and the arrow will leave the string behind. The string has to exit the nock groove. How it does this will effect the arrow rotation in the horizontal plane. The higher the bracing height then the earlier will the string exit the nock groove.
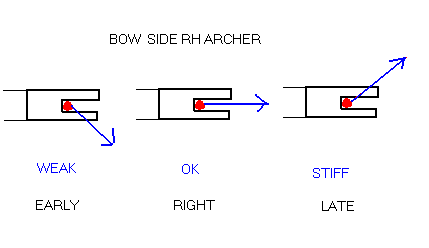The ideal situation is when the string direction of travel (the blue arrows) runs straight down the string groove. The string has no effect on
arrow rotation. If the brace height is too high the string will exit the
nock too early and the direction of string deceleration runs through 'left
hand side' of the nock groove. The consequent tweak on the arrow acts to
increase arrow rotation in a clockwise direction (looking down on the bow)
which makes the arrow act weak. If the brace height is too low you get the
reverse and the arrow acts stiff. Generally, because of the way the arrow
is flexing and moving, the consequences of having too late a string-nock
separation will be worse than having one too early.
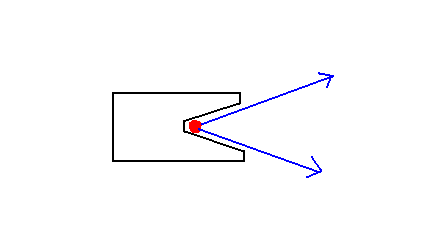The tighter the string fit in the nock then the worse will be the consequences
of the string not exiting the nock cleanly. The string-nock friction will
add to the amount of rotation the arrow gets. This is why the nocking point
should be just tight enough not to slide around in the nock groove during
the draw. As the diagram illustrates having a short 'V' shaped
nock provides a wider tolerance for the string to exit the nock cleanly.
In practice nocks are usually keyhole shape; the string is free to swivel inside the nock to prevent string/arrow torque while the "pinch" keeps the arrow from falling off the string during the draw process
The effect of bracing height on string-nock separation is not something that the archer need to be directly concerned with. When you select your arrow based on draw length and weight from the charts and use a bracing height in the recommended range this, by definition, gives you good nock-string separation. When you 'tune for groups' you are making small adjustments to how the arrow bends and rotates and getting the best nock-string separation is automatically included in the process.
If you make the assumptions that during the shot the time the arrow is on the bowstring equals the free-free shaft vibration time and that the arrow goes through 0.25 vibration cycles between the nock leaving the bow string and passing the pressure button then you get the relation that the bracing height equals the arrow launch kinetic energy divided by the peak draw force.
The following snapshots from Lloyd Brown's video of Park Sung-Hyun illustrate this idea.
At the point the arrow leaves the string, at around the bracing height, the shaft is bent around the riser. Lots of riser clearance available. The arrow at the
point of leaving the string has gone through three quarters of a flexing cycle (straight->bent->straight->bent). The lateral restraint on the nock by the string (the
limb torsional stiffness) slows the nominal free-free arrow vibration frequency by around 25%. It follows that the time the arrow is on the string equals the
time for the arrow to go through one free-free shaft vibration cycle. Using Newton's force equals rate change of momentum and assuming a linear string force
variation we get 1/f = 2mv/gw
where:
f is the free free vibration frequency
m is the arrow mass
v is the arrow launch speed
g is the gravitational acceleration
w is the bow draw weight
As the arrow moves forward off the string the back end of the shaft flexes away from the riser maintaining the clearance. At the point the fletchings pass
the riser the shaft is more or less straight.
To flex from bent to straight is one quarter (0.25) of a free-free vibration cycle. Using time equals distance divided by speed we get
1/f = 4b/v where
b is the bow bracing height.
It follows that for a correct arrow match to the bow meeting the Archers Paradox requirement (one flex cycle from launch to nock passing the riser) we have
the equality: 2mv/gw = 4b/v . i.e. rearranging; brace height times draw force equals the arrow kinetic energy
For example using data from Dennis Lieu
A tuned bow system with an ACC 3L-18 arrow had the following measured properties:
Arrow mass 341 grain (23.01 grams)
Draw Force 169N
Arrow launch speed 57.9 metres/second
Using the KE/Draw force method with the above data gives a bracing height requirement of 22.8 cms
The actual measured bracing height from the physically set up and tuned bow was 22.5 cms
The actual measured free-free vibration frequency (f) for the arrow was 68Hz with a calculated range of frequencies re clearance of 66-72Hz. The calculated frequencies based on the Archers Paradox requirement as above for the arrow on string and crossing the brace height are 63Hz and 64Hz respectively.
Not in itself particularly useful perhaps but rearranging the equation gives you an estimate of arrow speed based on arrow mass, draw force and brace height which might be useful as it only needs numbers that any archer can easily measure.
i.e. Arrow Speed squared = twice brace height times draw force divided by arrow mass
With the above example and the measured brace height of 22.5 cms the estimated arrow speed is 57.5 m/s.
Last Revision 4 April 2014