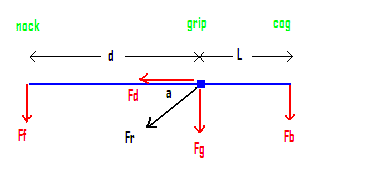
The diagram illustrates these three forces for the case where the gravity forces are at 90 degrees
to the draw force.There are three contributions to the force that the bow hand has to sustain, the force from drawing the bow, the gravity force on the bow and the balancing moment force exerted by the string hand.
The diagram illustrates these three forces for the case where the gravity forces are at 90 degrees
to the draw force.
Fb is the vertical gravity force on the bow mass which acts through the bow Centre of Gravity (cog).
Ff is the vertical force that has to be exerted by the string fingers on the bow to balance the moment
of the gravity force on the bow around the grip. Ff = Fb(L/d) where 'L' is the horizontal cog to grip
distance and 'd' is the nock to grip distance. The total vertical load on the bow hand
Fg = Fb+Ff so Fg = (1+L/d)Fb.
As the vertical (gravity) load on the bow hand depends on the ratio L/d this has some implications for the bow setup. If you move the centre of gravity of the bow forward you increase the load the bowhand is required to carry. For a given gravity load on the bow hand there is a relationship between the draw length and the COG position. As the draw length increases, then to keep the bow hand loading the same the COG has to move forward (e.g longer draw length = longer extender bar).
The total force that the bow hand has to support Fr is the vector sum of the draw force Fd and the gravity force Fg. The direction of
the force Fr (represented by angle 'a') is determined by the ratio Fg/Fd. i.e.
Tan(a) = (1+L/d)Fb/Fd.
In Archery Anatomy Axford discusses how the stability of the bow arm shoulder is affected by the value of the angle 'a'. This can now be expanded to include draw length and bow centre of gravity position as factors in shoulder stability.
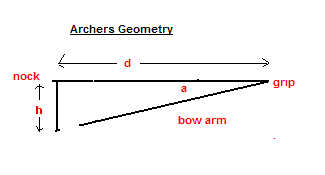From an archer's geometry point of view, if we assume as
previously that the nock to grip line is horizontal, then approximately
Tan(a) =h/d where 'h' is the vertical distance from the arrow nock to a line through the shoulders.
For the resultant force on the bow hand to run straight through the bow arm then you have the equality:
h/d = (Fb/Fd)(1+L/d)
The above equation gives some indication of how the various parameters affecting
bow arm stability interrelate.
To express how heavy a bow aproximately needs to be for good balance a "power to weight" ratio factor is sometimes used. That is you divide the draw weight by this ratio to esimate the bow weight. As the power to weight ratio is by definition Fd/Fb the above equation can be used to give this ratio from some simple values. e.g. if h = 4", L = 2.5" and d = 28" the power to weight ratio is about 7.6. So if your draw weight is 40 pounds then the bow needs to weigh around 40/7.6 = 5.25 pounds.
In reality the bow will be raised (or sometimes lowered) for actual shooting at distance or uphill/downhill and this will have an effect on the overall bow hand loading. As the bow is raised above horizontal the value of Fr will increase and the value of 'a' decrease and vice versa as the bow is lowered.
While the bow hand load running through the bow arm may seem to be the mechanically the best option as the shoulder muscles need to exert minimum effort to keep the bow hand stationary, biomechnically this may not be the best option. I know zip about biomechanics. I've heard coach opinion that the bow hand load should run above the shoulder and coach opinion that the bow hand load should run below the shoulder. Take your pick but either way the force direction will be close to the line of the bow arm so the above equation can be subjectively useful.
How in practice do you set up a stabiliser system to get a balanced recurve bow. The only method I know of is "feel". If the balance is correct then at full draw the bow should feel mass neutral in the vertical plane. There is no sensation of vertical weight acting downwards or upwards on the bow hand. The benefits to a balanced bow to aiming stability are obvious. Stabilisers are used to do three things at the same time; balancing the bow at the aiming stage, dynamically stabilising the bow during the bow power stroke and dumping vibrational energy to protect the archer. So how do you balance a bow while optimising the other two stabiliser functions. The answer is the V bar and twin rod system.
If you look at any high class recurve archer shooting line then almost universally the same stabiliser assembly is seen on each bow, an extender a flat V bar and twin system and long rod usually fitted with end weights. Why this arrangement?
Fletchings were used on arrows, purely because of the performance increase achieved, thousands of years before how fletchings worked was understood. To some extent I believe the almost universal recurve stabiliser arrangement is experience based rather than knowledge based. The long rod, V bar and twin rod arrangement is used mainly because it's what's found in practice to give the best results . I've never seen a comprehensive description of why the V bar/twin rod assembly is so successful and I don't claim to have all the answers either. What I'll try to do here is take a reverse engineering approach essentially giving my opinions as to what the V bar/twin rod approach is for and why it's better than any alternative. On occasion I'll have to bring in aspects relating to dynamic bow stabilisation covered elsewhere and I'm not going to repeat the explanations.
Nearly all V bar/twin rod arrangements have a vibration damping function. At one time they all used "torque flight compensators (TFCs)" some still do in the classic approach of a chunk of rubber between the twin rod and the V bar, some rods have the equivalent of TFCs built in, some use the carbon rod itself as a damper but probably the most popular approach today is some form of Doinker incorporated into the end weights.
The typical weight arrangement on twin rods is solidly fixed weights with very often a doinker type arrangement at the end. As regards vertical load on the bow hand (bow static balance) both fixed weights and doinker weights contribute. As regards dynamic stabilisation only the fixed weights contribute significantly. With any movement of the riser during the power stroke the doinker rubber flexes so any mass beyond the doinker contributes very little to bow stabilisation.
OK, so the V bar/twin rod arrangement is used for static bow balance, dynamic stabilisation and vibration energy dumping. Is there an order of importance for these three functions. There are clearly simpler alternatives as regards vibration damping (e.g.doinkers in top/bottom risers bushings) so that is probably not it's primary function. As regards dynamic stabilisation while the twin rod weights make a significant contribution (around a vertical axis through the grip much greater than that from the riser) it is small compared to what you get from the long rod. The suggested conclusion is that the primary function of the V bar twin assembly is static bow balance.
The primary function of the long rod is dynamic bow stabilisation by increasing bow moi and pushing the bow centre of mass forwards of the grip. The limit on this is rod stiffness. We use an extender to effectively increase rod length while maintaining rod stiffness. The break between extender and long rod provides a convenient point to insert a V bar to carry a twin rod system. Now for the leap of faith (or my guess if you will). When the long rod system on a recurve bow is maximised there is insufficient vertical mass load on the bow hand to statically balance the bow.
Riser manufacturers don't know in advance what draw length and what draw weight any particular user is going to have so they have to make the riser light enough to cater for everybody. So when the archer has maximised the dynamic stabilisation with the long rod/extender additional mass is required for static bow balance. How do you add this mass. A consideration here is the overall force on the bow hand. The more mass we add the higher the force on the bow hand. So another rule is that we want to minimise the amount of mass added to the bow to achieve static bow balance in order to minimise the force on the bow hand.
In order to minimise the amount of mass added to balance a bow the mass needs to placed as close as possible in a vertical plane running through the bow pivot point (grip) at 90 degrees to the plane of the bow. The reason why is explained above with regard to the balancing moment required for bow centre of mass. Place extra mass anywhere else and the additional vertical bow hand load is greater than the physically added mass. When we shoot an arrow the riser accelerates away from the archer in a horizontal plane. To increase lateral stability we want mass in the horizontal plane of the grip at 90 degrees to the bow plane.. For We don't want to place the twin weights in front of the grip as this would increase the stress on the extender with respect to rotation about a horizontal axis (the major load on the extender presumably already maximised with respect to long rod dynamic stabilisation). Having the twin weights in front of the grip will also reduce the weight to grip distance unless you increase the V bar angle.I once tried reversing the V bar so the twin rods ran forwards. The result was hopeless as my (admittedly cheap) extender just flexed during the power stroke. The stabilisation system just went "mushy". We don't want to place the twin rod weights to the rear of the grip as this eats away at the dynamic stabilisation from the long rod (reduced gravity torque). By putting the twin weights in the horizontal axis through the bow pivot point at 90 degrees to the bow plane we can add as much weight as we like without penalty to the dynamic stabilisation of the bow from the long rod.
There are a number of solutions to how to add this extra mass. Adding mass directly to the riser is a possibility (screw in weights?), using a back weight attached to the bow is a used solution and using a V bar/twin rod system to add weights in the correct plane is the common solution. The down side to the back weight is that although in addition to achieving static bow balance it marginally adds to the bow moi about the grip being the wrong side of the pivot it reduces the gravity torque on the bow. The main advantage of the back weight is it creates more space on the shooting line. The advantages of the V bar/twin arrangement become clear.
The last point is the detail of the extender and twin geometry. The norm is a flat or slightly down angled V bar system with a 90 degree V bar angle. Why?
Too small a V bar angle and the rods start getting in the way; too large an angle and the rods get too long and wide for convenience or you have to shorten the extender which impacts on the dynamic stabilisation; angling the rods down has little impact on lowering the bow cog and you have the same issues as too large a V bar angle. Angling the twin rods upwards has the effect of marginally raising the bow centre of gravity which is never a good idea as regards dynamic stabilisation.And so on. By experience the "standard geometry" gives you all round the best solution.
Last Revision 1 July 2009